![]()
![]()
![]()
Use LEFT and RIGHT arrow keys to navigate between flashcards;
Use UP and DOWN arrow keys to flip the card;
H to show hint;
A reads text to speech;
11 Cards in this Set
- Front
- Back
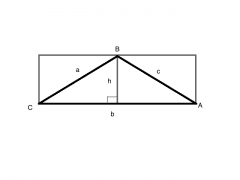
Given acute ∆ABC with a, b, c, being respective opposite sides to angle A, angle B, angle C, and altitude h, drawn from angle B to b, prove that the area of ∆ ABC = 1/2 a*b*sin(C)
|
∆ ABC is drawn inside a rectangle where the altitude, h, is perpindicular to base b. The area of the rectangle is hb and the area of the triangle must be 1/2hb since it neatly divides the rectangle into two pairs of congruent triangles. We know that the length h can be found using the sine of angle C where sin C=h/a or a*sin C=h. Therefore, 1/2hb= 1/2*a*sin C*b, or area=1/2*a*b*sinC
|
|
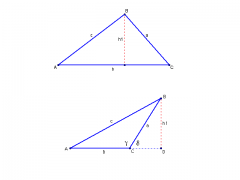
Given ∆ABC, prove that a/sin A= b/sin B= c/sin C
|
Begin by constructing altitude h from vertex B to side b in an acute triangle (in an obtuse triangle, extend side AC and construct the altitue from vertex B). Note that sin A= h/c, therefore h=c*sin A. Similarly, sin C= h/a, therefore h= a*sin C. Now we know that c*sin A= a*sin C, which means a/sin A= c/sin C. Similar reasoning can be used to show that a/sin A= b/sin B and c/sin C= b/sin B. Therefore we have demonstrated a/sin A= b/sin B= c/sin C.
|
|

Given acute ∆ABC, prove that c²=a²+b²-2abcos(C)
|
∆ABC. We drop a perpendicular from point B to intersect with side AC at point D, which creates 2 right triangles (ABD and CBD). Side b from ∆ABC is equal to side d from ∆ABD plus side e from ∆CBD. Since ∆ABD and ∆CBD are both right triangles, we can use the Pythagorean Formula to identify the relationship between the hypotenuse and each leg of the triangle. For ∆ABD, we get c²=d²+h². Since b=d+e, this means d=b-e. By substitution, c=(b-e)²+h². Since we also know that sin(C) in ∆CBD=h/a, we know that h=a*sin(C). By substitution, c²=(b-e)²+(a*sin(C))²= (b-e)²+a²*sin²(c). We know that (b-e)²=b²-2*b*e*e². By substitution, c²=b²-2*b*e+e²+a²*sin²(C). We know that cos(C) in ∆CBD is equal to e/a and therefore e=a*cos(C). By substitution, c²=b²-2*b*a*cos(C)+(a*cos(C))²+a²+sin²(C)=b²-2*b*a*cos(C)+(a²*cos²(C))+(a²*sin²(C)). By the commutative property, b*a=a*b. We can factor out the a² and we end up with c²=b²-2*a*b*cos(C)+a²(cos²(C))+sin²(C)). Since we know that cos²(C)+sin²(C)=1, by substitution c²=b²-2*a*b*cos(C)+a². Rearranging terms, this becomes c²=a²+b²-2*a*b*cos(C)
|
|
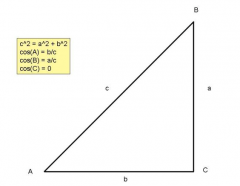
Given right triangle ABC, prove that c²=a²+b²-2abcos(C)
|
Since triangle ABC is a right triangle, the Pythagorean Formula prevails. We start off with our original equation of c²=a²+b²-2*a*b*cos(C). since c is the hypotenuse of this right triangle, then angle C is opposite side c and is equal to 90°. If angle C is equal to 90°, then cos(C)=cos(90°)=0. This makes 2*a*b*cos(C) equal to 0. The equation c²=a²+b²-2*a*b*cos(C) becomes c²=a²+b² which is exactly the Pythagorean formula. Since the Pythagorean formula prevails in a right triangle, and the Pythagorean Formula is a special case of our original equation, we are done.
|
|
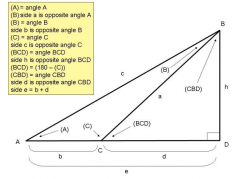
Given obtuse triangle ABC, prove that c²=a²+b²-2abcos(C)
|
In this case, ∆ABC is obtuse where angle C is the obtuse angle. We drop a perpendicular from point B to intersect with the extension of side b at point D. We are trying to prove that c²=a²+b²-2*a*b*cos(C). We start off with c²=e²+h² from the right ∆ABD in the triangle. This is from the well known Pythagorean Formula of c²=a²+b² where, in our triangle, c=c, e=a and h=b. From our diagram, we can see that sin(BCD)=h/a or h=a*sin(BCD). By substitution, c²=e²+(a*sin(BCD))². Since we know that the sine of the supplement of an angle is the same as the sine of the angle, we can substitute (C) for (BCD) to get c²=e²+(a*sin(C))². Since we know that (a*sin(C))² is the same as a²*sin²(C), by substitution, c²=e²+a²*sin²(C). Since we can see from the diagram that e=b+d, by substitution, c²=(b+d)²+a²*sin²(C). Since we know that (b+d)²=b²+2*b*d+d², by substitution, c²=b²+2*b*d+d²+a²*sin²(C). Since we know that cos(BCD)=d/a, and from that we can derive d=a*cos(BCD), by substitution,c²=b²+2*b*a*-cos(C)+(a*(-cos(C)))²+a²*sin²(C). Since we know that 2*b*a*(-cos(C))=-2*a*b*cos(C), and since we know that (a*(-cos(C)))² is the same as a²*cos(C)², then by substitution, c²=b²-2*a*b*cos(C)+a²*cos²(C)+a²*sin²(C). Since we know that a²*cos²(C)+a²*sin²(C)=a²*(cos²(C)+sin²(C)) and since we know that cos²(C)+sin²(C)=1, then we can substitute a² for a²*cos²(C)+a²*sin²(C) in our equation to get c²+b²-2*a*b*cos(C)+a², and then rearrange the terms to get c²=a²+b²-2*a*b*cos(C)
|
|
|
In Triangle XYZ, sin X=16, sin Y=0.4 and y=17. find the length of X using the law of sines.
|
x/sin(X)= y/sin(Y)
x/sin(16)=17/sin(0.4) x*sin(0.4)= 17*sin(16) x*0.007=17*0.2756 (17*0.2756)/0.007=x x=671.20 |
|
|
In triangle PQR, side q=11, m<Q=74° and m<R=63°. Find side r to the nearest tenth of an integer.
|
q/sin(Q)=r/sin(R)
11/sin(74)=r/sin(63) r*sin(74)=11*sin(63) r*0.9613=11*0.8910 r=(11*0.8910)/0.9613 r=10.196 |
|
|
In triangle ABC, a=8, c=17 and m<A=40d. How many distinct triangles can be drawn given these measurements?
|
Use the law of sines a/sin(A)=c/sin(C) 8/sin(40d)=17/sin(C)
8(sin(C))=17*sin(40d) sin(C)=17*0.6/8=1.275 since sin C must be <= 1, no angle exists for angle C. No triangle exists for these measurements. |
|
|
In triangle ABC, a=12, b=17, and m<A=20d. How many distinct triangles can be drawn given these measurements?
|
Use the law of sines a/sin(A)=b/sin(B)
12/sin(20d)=17/sin(B) 12(sin(B))=17(sin(20d)) sin(B)=17*0.3/12= 0.425 Angles could be 20°, 25.15°, and 134.85°: sum 180°. Now m<A=20 and m<B=25.15 the sum of the angles would exceed 180d. Not possible. Therefore, m<B=25.15, m<A=2-, and m<C=134.85d and only ONE triangle is possible. |
|
|
Find the missing angle given the following information: BC=10; AC=18; AB=7 Sin_____=7/18
|
sin(x)=7/18
sin(x)=0.4 x=sin⁻¹(0.4) x=23.6d |
|
|
Find the missing angle given the following information: BC=24; AC=13; AB=4 tan_____=13/24
|
tan(x)=13/24
tan(x)=0.5417 x=tan⁻¹(0.5417) x=26.6° |

