![]()
![]()
![]()
Use LEFT and RIGHT arrow keys to navigate between flashcards;
Use UP and DOWN arrow keys to flip the card;
H to show hint;
A reads text to speech;
12 Cards in this Set
- Front
- Back
|
Prove or disprove that a figure defined by the points A (2,4), B (4,2), C (8,6), and D (6,8) in the coordinate plane is a rectangle.
|
1. ABCD is a quadrilateral. 1a. A quadrilateral is a four-sided figure. 2. The slope of AB is -1. 2a. ∆y/∆x= (2-4)/(4-2) ∆y/∆x= -2/2 ∆y/∆x=-1. 3. The slope of DC is -1. 3a. ∆y/∆x=(6-8)/(8-6) ∆y/∆x= -2/2 ∆y/∆x=-1. 4. AB ‖ CD. 4a. Parallel lines have the same slope. 5. The slope of BC is 1. 5a. ∆y/∆x= (6-2)/(8-4) ∆y/∆x= 4/4 ∆y/∆x=1. 6. The slope of AD is 1. 6a. ∆y/∆x= (8-4)/(6-2) ∆y/∆x=4/4 ∆y/∆x=1. 7. BC ‖ AD. 7a. Parallel lines have the same slope. 8. ABCD is a parallelogram. 8a. A parallelogram is a quadrilateral with two pairs of parallel sides. 9. AB ⊥ BC. 9a. Perpendicular lines have inverse slopes. 10. BC ⊥ DC. 10a. Perpendicular lines have inverse slopes. 11. DC ⊥ AD. 11a. Perpendicular lines have inverse slopes. 12. DA ⊥ AB. 12a. Perpendicular lines have inverse slopes. 13. ∠ABC, ∠BCD, ∠CDA, and ∠DAB are right angles. 13a. Perpendicular lines form right angles. 14. ABCD is a rectangle. 14a. A rectangle is a parallelogram with 4 right angles.
|
|
|
Prove or disprove that the point (1,√3) lies on the circle centered at the origin and containing the point (0,2)
|
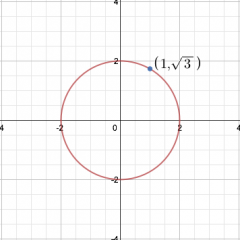
First, determine the equation for the circle: x²+y²=2². Next, insert the coordinates for the point in question (1,√3) into the equation. If the coordinates satisfy the equation, then the point must lie on the circle. x²+y²=2² (1)²+(√3)2=2² 1+3=4 4=4, therefore (1,√3) must lie on the circle centered at the origin and containing the point (0,2).
|
|
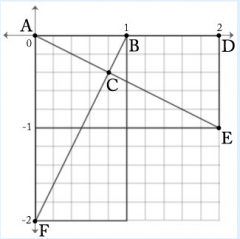
Three unit squares and two line segments connecting two pairs of vertices are shown. What is the area of ∆ ABC? (hint: use the coordinate system to help you solve this problem.)
|

Using AB as a base, the altitude of the triangle is 2/5 since, as we can see on the coordinate plane, C is 2/5 units below the x-axis. A=1/2bh A=(1/2)(1)(2/5) A=1/5 sq units
|
|
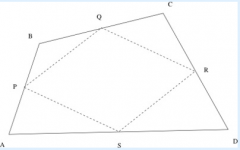
Given is quadrilateral ABCD, with no congruent sides or angles, and no two sides parallel. P, Q, R, and S are the midpoints of sides AB, BC, CD, and DA, respectively. What do you notice about the quadrilateral PQRS?
|
PQRS appears to be a parallelogram.
|
|
|
Suppose quadrilateral ABCD lies in the coordinate plane. Let (x₁,y₁) be the coordinates of vertex A, (x₂,y₂) the coordinates of B, (x₃,y₃) the coordinates of C, and (x₄,y₄) the coordinates of D. Use coordinates to prove that PQRS is a parallelogram.
|
1. Coordinates of P are (x₁+x₂/2,y₁+y₂/2). 1a. P is half way between points A (x₁,y₁) and B (x₂,y₂). 2. Coordinates of Q are (x₂+x₃/2,y₂+y₃/2). 2a. Q is half way between points B (x₂,y₂) and C (x₃,y₃). 3. Coordinates of R are (x₃+x₄/2,y₃+y₄/2). 3a. R is half way between points C (x₃,y₃) and D (x₄,y₄). 4. Coordinates of S are (x₄+x₁/2,y₄+y₁/2). 4a. S is half way between points D (x₄,y₄) and A (x₁,y₁). 5. The slope of PQ is y₃-y₁/x₃-x₁. 5a. ∆y/∆x= (y₂+y₃/2 - y₁+y₂/2)/(x₂+x₃/2 - x₁+x₂/2) ∆y/∆x= (y₂+y₃)-(y₁+y₂)/(x₂+x₃)-(x₁+x₂) ∆y/∆x= y₃-y₁/x₃-x₁. 6. The slope of SR is y₃-y₁/x₃-x₁. 6a. ∆y/∆x=(y₃+y₄/2 - y₄+y₁/2)/(x₃+x₄/2 - x₄+x₁/2) ∆y/∆x= (y₃+y₄)-(y₄+y₁)/(x₃+x₄)-(x₄+x₁) ∆y/∆x= y₃-y₁/x₃-x₁. 7. PQ ‖ SR. 7a. Parallel lines have the same slope. 8. The slope of QR is y₄-y₂/x₄-x₂. 8a. ∆y/∆x= (y₃+y₄/2 - y₂+y₃/2)/(x₃+x₄/2 - x₂+x₃/2) ∆y/∆x= (y₃+y₄)-(y₂+y₃)/(x₃+x₄)-(x₂+x₃) ∆y/∆x= y₄-y₂/x₄-x₂. 9. The slope of PS is y₄-y₂/x₄-x₂. 9a. ∆y/∆x= (y₄+y₁/2 - y₁+y₂/2)/(x₄+x₁/2 - x₁+x₂/2) ∆y/∆x= (y₄+y₁)-(y₁+y₂)/(x₄+x₁)-(x₁+x₂) ∆y/∆x= y₄-y₂/x₄-x₂. 10 QR ‖ PS. 10a. Parallel lines have the same slope. 11. PQRS is a parallelogram. 11a. Parallelograms have two pairs of parallel sides.
|
|
|
Find the equation of a line parallel to y=1/2x+3 that passes through (1,9).
|
y= 1/2x+3
y=1/2x+b (9)=1/2(1)+b 9=1/2+b b=8 1/2 y=1/2x+8 1/2 |
|
|
Find the equation of a line perpendicular to y=3x-1 that passes through (4,14).
|
y=3x-1
y=3x+b (14)=3(4)+b 14=12+b b=2 y=3x+2 |
|
|
Find a point on line segment AB between points A (-2,-1) and B (4,7) that partitions the segment into two parts whose ratio is 1:1
|
A point that partitions the segment into two parts whose ratio is 1:1 is a midpoint. The formula to find a midpoint is M=(x1+x2/2,y1+y2/2)
M=(-2+4/2, -1+7/2) M=(2/2, 6/2) M=(1,3) |
|
|
Find a point on line segment AB between points A (-5,6) and B (1,3) that partitions the line into two parts whose ratio is 3:1
|
A point that partitions the segment into two parts whose ration is 3:1 divides the line into 4 equal parts, 3 of which fall on one side of the point, and 1 of which falls on the other side of the point. We can find this point by finding the midpoint of the line segment, C, then the midpoint of the smaller line segment, CB.
C=(x1+x2/2,y1+y2/2) C=(-5+1/2,6+3/2) C=(-4/2,9/2) C=(-2,4/5) M=(x1+x2/2,y1+y2/2) M=(-2+1/2,5+3/2) M=(-1/2,8/2) M=(-1/2, 4) The point that partitions the line segment into two parts whose ratio is 3:1 is (-1/2,4) |
|
|
Given the coordinates of pentagon ABCDE, use the distance formula to find its perimeter. A (-5,3), B(0,4), C(4,2), D(2,-3), and E(-4,-3)
|
In order to find the perimeter, we must calculate the length of each side (line segment) and then add them together. The distance formula will be used to find the length of each side. d=S√(x₂-x₁)²+(y₂-y₁)²
Side AB: d=√(0-(-5))²+(4-3)² d=√26 d≈5.1 Side BC: d=√(4-0)²+(2-4)² d=√20 d≈4.5 Side CD: d=√(2-4)²+(-3-2)² d=√29 d≈5.4 Side DE: d=√(-4-2)²+(-3-(-3))² d=√36 d=6 Side EA: d=√(-5-(-4))²+(3-(-3)² d=√1 d=1 Next, we must add the values together: AB+BC+CD+DE+EA= 5.1+4.5+5.4+6+1=22 The approximate perimeter of ABCDE is 22 units. |
|
|
Given the coordinates of right triangle QRS, find its area. Q(0,1) R(1,5) S(0,4) Hint: Use the distance formula to find the side lengths.
|
To find the area of right triangle QRS, first we must calculate the side lengths. Then, we must determine which sides are the base and height, and input them into the area formula A=1/2bh. To find the side lengths, the distance formula will be used.
d=√(x₂-x₁)²+(y₂-y₁)² Side QR: d=√(1-0)²-(5-1)² d=√17 Side RS: d=√(0-1)²+(4-5)² d=√2 Side SQ: d=√(0-0)²+(1-4)² d=√9 d=3 Since side QR is the longest, it is the hypotenuse. We will use sides RS and SQ to find the area. A=1/2bh A=1/2(√2)(3) A=2.1 The approximate area of QRS is 2.1 square units. |
|
|
Given the coordinates of rectangle ABCD, find its area. A(-1,4), B(-1,0), C(6,0), and D(6,4) Hint: use the distance formula to find the side lengths.
|
To find the area of the rectangle we need to find two side lengths that are not the same. We will compute the length of sides AB and BC using the distance formula, and then input the values into the area formula A=bh. Side AB: d=√(x₂-x₁)+(y₂-y₁)
d=√(-1-(-1))²+(0-4)² d=√16 d=4 Side BC: d=√(6-(-1))²+(0-0)² d=√49 d=7 A=bh A=(4)(7) A=28 square units. |

