Geometry Chapter 3 Theorems, Postulates, Definitions

Front 1

Definition of Skew Lines
3-1

Back 1

If two lines are skew, then they do not intersect and are not in the same plane.

Front 2

Parallel Lines
3-1

Back 2

If two (or more) lines in a plane never intersect, then they are parallel.

Front 3

Corresponding Angles Postulate
3-2

Back 3

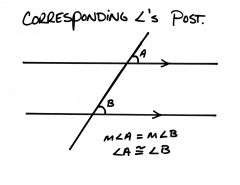
If two parallel lines are cut by a transversal, then each pair of corresponding angles is congruent.

Front 4

Alternate Interior Angles Theorem
3-2

Back 4

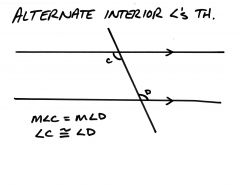
If two parallel lines are cut by a transversal, then each pair of alternate interior angles is congruent

Front 5

Consecutive Interior Angles Theorem
3-2

Back 5

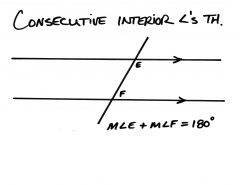
If two parallel lines are cut by a transversal, then each pair of consecutive interior angels is supplementary.

Front 6

Alternate Exterior Angles Theorem
3-2

Back 6

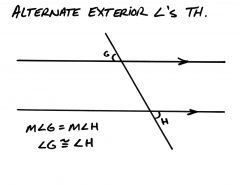
If two parallel lines are cut by a transversal, then each pair of alternate exterior angles is congruent

Front 7

Perpendicular Transversal Theorem
3-2

Back 7

In a plane, if a line is perpendicular to one of two parallel lines, then it is perpendicular to the other.

Front 8

Definition of Slope
3-3

Back 8

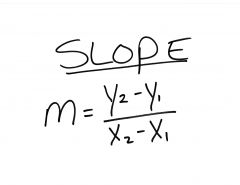
The slope m of a line containing two points with coordinates (X1, Y1) and
(X2, Y2) is given by the formula
m = Y2 - Y1 / X2 - X1

Front 9

Parallel Lines Postulate
3-3

Back 9

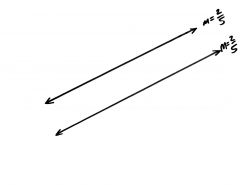
Two non-vertical lines have the same slope if and only if they are parallel.

Front 10

Perpendicular Lines Postulate
3-3

Back 10

Two non-vertical lines are perpendicular if and only if the product of their slopes is -1.

Front 11

Converse of the Corresponding Angles Postulate
3-4

Back 11

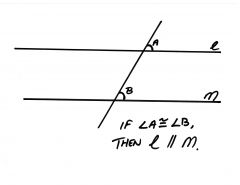
If two lines in a plane are cut by a transversal so that corresponding angles are congruent, then the lines are parallel.

Front 12

Parallel Postulate
3-4

Back 12

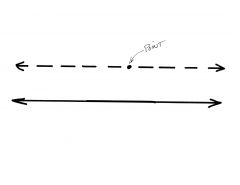
If there is a line and a point not on the line, then there exists exactly one line through the point that is parallel to the given line.

Front 13

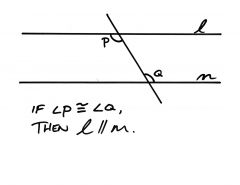
Converse of the Alternative Angles Theorem
3-4

Back 13

If two lines in a plane are cut by a transversal so that a pair of alternate exterior angles are congruent, then the two lines are parallel.

Front 14

Converse of the Consecutive Interior Angles Theorem
3-4

Back 14

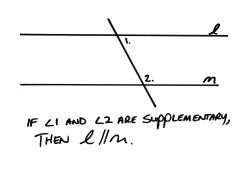
If two lines in a plane are cut by a transversal so that a pair of consecutive interior angles is supplementary, then the lines are parallel.

Front 15

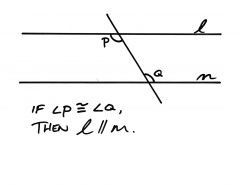
Converse of the Alternate Interior Angles Theorem
3-4

Back 15

If two lines in a plane are cut by a transversal so that a pair of alternate interior angles is congruent, then the lines are parallel.

Front 16

Converse of the Perpendicular Transversal Theorem
3-4

Back 16

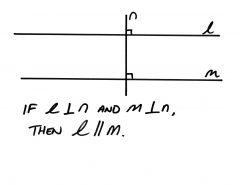
In a plane, if two lines are perpendicular to the same line, then they are parallel.

Front 17

Definition of the Distance Between a Point and a Line
(Not on the test) 3-5

Back 17

The distance from a line to a point not on the line is the length of the segment perpendicular to the line from the point.

Front 18

Definition of the Distance Between Parallel Lines
(Not on the test) 3-5

Back 18

The distance between two parallel lines is the distance between one of the lines and any point on the other line.