![]()
![]()
![]()
Use LEFT and RIGHT arrow keys to navigate between flashcards;
Use UP and DOWN arrow keys to flip the card;
H to show hint;
A reads text to speech;
14 Cards in this Set
- Front
- Back
|
What is the formula for the Pythagorean theorem?
|
a²+b²=c²
|
|
|
In the formula for the Pythagorean theorem, what do a, b and c stand for?
|
c stands for the length of the hypotenuse and a and b stand for the lengths of the two sides which are not the hypotenuse.
|
|
|
What is the hypotenuse of a right triangle?
|
The hypotenuse of a right triangle is the side opposite the right angle.
|
|
|
The Pythagorean Theorem states that: "In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides." Explain this relationship using sentences and a diagram.
|
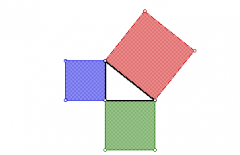
The Pythagorean Theorem is stating that a square created using the hypotenuse of a right angle as the side length will have an area equal to that of the sum of the area of the two squares created using the legs as their side lengths, as shown in this diagram. If the side length of the green square is a, the side length of the blue square is b and the side length of the red square is c, then, according to the theorem, a²+b²=c². This relationship has been proven in many ways both historically and in modern mathematics.
|
|
|
The Pythagorean Theorem is so named because ancient philosopher and mathematician Pythagoras was the first person to prove it. The theorem states that: "In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides" and is stated in the formula a²+b²=c². Explain Pythagoras' proof of the Pythaogrean Theorem using sentences and diagrams as necessary.
|
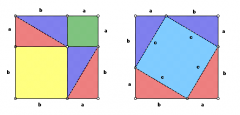
Pythagoras proved the theorem using squares, which are both shown in the diagram. The first square is divided into two equal-sized rectangles and two smaller squares, as shown in the diagram. The rectangles are then split into two equal right triangles by drawing the diagonal c. The two squares are not equal-sized. One has a side length of a, and the other has a side length of b. These four right triangles can be arranged differently within a square so that it has a side length of a+b, which is shown in the diagram as the second square. Both squares now have a side length of a+b so their areas must be equal. The area of the first square can be shown as the sum of the area of the two rectangles and the two squares, as A= a²+b²+2ab. The area of the second square can be shown as the sum of the areas of the square and the four triangles, as A= c²+ 4(ab/2) or A=c²+2ab. Since we know the areas of the squares are equal, we can set the two equations equal, as a²+b²+2ab=c²+2ab. Simplified, this expression is a²+b²=c², which proves that the squre on the hypotenuse (c) is equal to the sum of the squares on the legs (a and b).
|
|
|
The Pythagorean Theorem states that: "In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides" and is stated in the formula a²+b²=c². Explain Bhaskara's first proof of the Pythaogrean Theorem using sentences and diagrams as necessary.
|
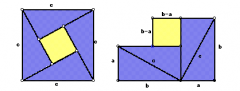
Bhaskara's first proof is similar to Pythagoras' proof. He used the two shapes shown in the diagram and the area formula to prove the theorem. In the diagrams, the right triangles (blue) are all congruent and the yellow squares are congruent. Bhaskara showed that the area of the big square can be found two different ways. It can be found by squaring the side length (c) of the square, as A=c² or it can be found by adding the areas of the triangles to the area of the small square as shown in the second shape in the diagram, as A= 4(1/2)ab + (b-a)². Bhaskara then simplified the second expression as such: A= 4(1/2)ab + (b-a)² = 2ab + b² - 2ab + a² = b²+a². Since the areas must be equal, Bhaskara concluded the proof by stating that c²=a²+b²
|
|
|
The Pythagorean Theorem states that: "In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides" and is stated in the formula a²+b²=c². Explain Bhaskara's second proof of the Pythaogrean Theorem using sentences and diagrams as necessary.
|
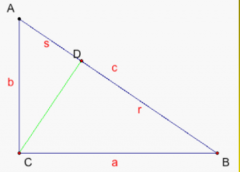
Bhaskara's second proof relies on the properties of similarity to prove the Pythagorean Theorem. He began with a right triangle, ABC as shown in the diagram, and then drew an altitude to the hypotenuse and stated that the 2 triangles formed are similar to the original triangle and to each other, so all 3 triangles are similar. First, he proved that triangles ADC and ABC are similar using the angle-angle principle of similarity, as such: angle DBC is congruent to angle ABC and angle ACB is congruent to angle ADC. Since the triangles are similar, the ratios of their side lengths must be equal, which can be shown as s/a=a/c. Multiplying both sides by ac, we get sc=a². Next, he proved that triangles ACD and ABC are similar, also using the angle-angle principle of similarity, as such: angle CAD is congruent to angle CAB and angle ADC is congruent to angle ACB. Since the triangles as similar, the ratios of their side lengths must be equal, which can be shown as r/b=b/c. Multiplying both sides by bc, we get rc=b². Now when we add the two results, we get sc+rc= a²+b². This expression can be simplified as such, noting as shown in the diagram that c=(s+r): c(s+r)=a²+b² c²=a²+b², which proves the Pythagorean Theorem.
|
|
|
The Pythagorean Theorem states that: "In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides" and is stated in the formula a²+b²=c². Another person to prove this theorem was the twentieth president of the United States, James Garfield. Explain Garfield's proof of the Pythaogrean Theorem using sentences and diagrams as necessary.
|
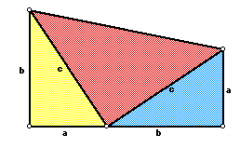
President Garfield used a right trapezoid, composed of three right triangles to prove the Pythagorean theorem. This trapezoid is shown in the diagram. He also used the area formula in his proof. Garfield said that the area of the trapezoid could be found in two ways, by using the area formula for a trapezoid and also by adding up the areas of the three right triangles that compose the trapezoid. First, he showed that the area of a trapezoid with h=a+b, b₁=a and b₂=b can be found as such: A=(1/2)(a+b)(a+b). Using the distributive property, this can be expressed as: A=(1/2)(a²+2ab+b²). Next, he showed that the area of the area of the trapezoid could be found by adding up the areas of the three right triangles as such A= area of yellow triangle + area of red triangle + area of blue triangle = 1/2(ba) + 1/2(c²) +1/2(ab). This can be simplified as such:1/2(ba) + 1/2(c²) + 1/2(ab) = 1/2(ba+c²+ab) = 1/2(2ab+c²). He then set the two methods of finding the area of the trapezoid equal to each other as (1/2)(a²+2ab+b²) = (1/2)(2ab+c²) and then multiplied both sides by 2, expressed as a²+2ab+b²=2ab+c². Finally, he subtracted 2b from both sides, which brings us to a²+b²=c², which proves the Pythagorean theorem.
|
|
|
Find the hypotenuse of an equilateral triangle with a base of 11 cm and a height of 9 cm. Round your answer to the nearest tenth.
|
a²+b²=c²
(11 cm)² + (9 cm)² = c² 121 cm² + 81 cm² = c² 202 cm² = c² √202 cm²= √c² 14.2 cm=c |
|
|
Tommy is painting his house. The bottom of a ladder is placed 6 feet from the house. The ladder is 10 feet long. How far above the ground will the ladder touch the house?
|
a²+b²=c²
a² + (6 ft)² = (10 ft)² a² + 36 ft² = 100 ft² a²= 64 √a²= √64 ft² a=8 ft |
|
|
Mary walked 3km west and 4km south to get to school. How far away is her house from the school if a straight line were drawn between the two?
|
a²+b²=c²
(3 km)²+(4 km)²=c² 9 km²+16 km²=c² 25 km²=c² √25 km² = √c² 5 km = c |
|
|
Apply the Pythagorean Theorem to find the distance between the two points.
|
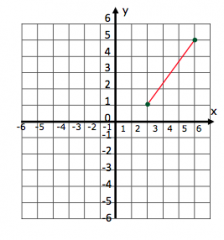
a²+b²=c²
3²+4²=c² 9+16=c² 25=c² √25 = √c² 5 = c |
|
|
Apply the Pythagorean Theorem to find the distance between the two points. Round your answer to the nearest tenth.
|
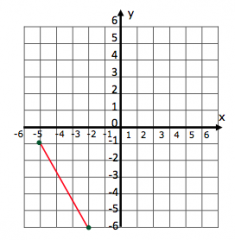
a²+b²=c²
3²+5² = c² 9+25=c² 34=c² √34 = √c² 5.8=c |
|
|
Apply the Pythagorean Theorem to find the distance between the two points. Round your answer to the nearest tenth.
|
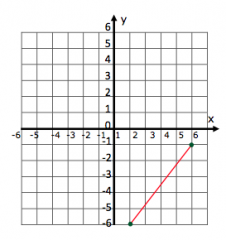
a²+b²=c²
4² + 5² = c² 16+25=c² 41=c² √41 = √c² 6.4=c |

