10/10-17, 201Q

Front 1

What proves particles are a wave?

Back 1

double slit experiment

Front 2

What proves light is a particle

Back 2

photoelectric effect

Front 3

Defining property of a wave

Back 3

a wave can be at the same point in space and time

Front 4

Energy of a photon

Back 4

E=hv (v = greek letter nu)

Front 5

What is ?Planck's constant

Back 5

h=6.626x10-34Js

Front 6

Equation for de Broglie wavelength

Back 6

Front 7

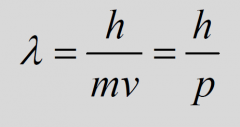
What describes the wave nature of a particle

Front 8

What is a wavefunction Wavefunction ψ

Back 8

function contains all the in formation about a system defined by the Schrodinger equation

Front 9

What is the Schrodinger equation

Back 9

Front 10

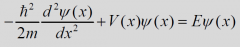
What is the kinetic energy equation

Back 10

e=1/2mv²

Front 11

What is Heisenberg's uncertainty principle equation

Back 11

Front 12

What is the usual Hamiltonian operator

Back 12

Front 13

What is an Operator

Back 13

Mathematical operation which is performed on a function

Front 14

How do we get quantised kinetic energy

Back 14

Take d²/dx² of wavefunction and multiply by -ℏ²/2m

Front 15

What makes up the total energy of a system.

Back 15

curvature of wavefunction multiplied by -ℏ²/2m

the potential energy of the system multiplied by the wavefunction

Front 16

What does the behaviour of curvature of the wavefuction depend on?

Back 16

Whether V(x)> E or V(x) < E

Front 17

How is magnitude of the curvature defined?

Back 17

(V(x) -E) or kinetec energy

Front 18

Where is the normal regime?

Back 18

where V(x) < E

Front 19

Where is the classically forbidden regime?

Back 19

V(x) > E

Front 20

When V(x)< E and the wavefunction is positive what is the curvature?

Back 20

negative (curves towards the x axis)

Front 21

When V(x)

Back 21

Positive (curves towards the x axis)

Front 22

When V(x) = 0, what does this mean?

Back 22

particle or wave is moving under no external forces

Front 23

When V(x) >E, and wavefuction is negative what is the curvature?

Back 23

negative(curves away from x axis)

Front 24

When V(x) >E, and wavefuction is positive what is the curvature?

Back 24

positive (curves away from x axis)

Front 25

What restrictions must a wavefunction must adhere to

Back 25

Must me continuous

single valued

Have a continuous derivative

Front 26

How do we get probability of finding a particle in space from wavefuction

Back 26

Intergrate the square of the modulus of the wavefunction between two points. (modulus because the square of complex numbers can be negative) ∫|ψ|² dx

Front 27

∫|ψ|² dx between ±∞ does not equal 1 what do we do?

Back 27

we normalise it (set it equal to 1)

Front 28

Units of probability?

Back 28

Unitless

Front 29

what are the units of ψ(x)? and why?
Edit: for one dimensional etc

Back 29

Front 30

What is the kinetic energy and momentum of a free particle where V(x) = 0.

Back 30

Ek = ℏ²k²/2m, momuntum is defined exactly as p=ℏk with k = 2π/λ

Front 31

What is the problem with knowing the momuntum of a particle exactly?

Back 31

You can't know anything about its position

Front 32

What does the superposition principle do?

Back 32

Allows us to localise a particle by linear combination of multiple wavefunction?

Front 33

How does the superposition principle work?

Back 33

Linear combination of multiple wavefunctions with different momentum values gives uncertainty in momentum meaning we should know more about its position (uncertainty principle), this is because different waves interfere giving localised regions where they are more likely to be than others

Front 34

What is the basis set?

Back 34

Set of wavefunctions that can be linearly combined denoted ψi

Front 35

If there is more than one solution to schrodinger equation for a particular situation what do we do?

Back 35

true wavefunction will be the linear combination of all possible functions

Front 36

Definitions of potential for Particle in a box system?

Back 36

V(x) =0 inside the box

V(x) = ∞ outside the box

Front 37

How do we write schrodinger equation for a particle in a box?

Back 37

Front 38

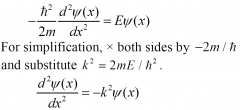
What potential solutions to the schrodinger equation for a particle in the box are there?

Back 38

Asin(kx) or Bcos(kx)

Front 39

What are the second derivatives of the two possible wavefunctions?

Back 39

d²/dx²(Asin(kx)) = -k²(Asin(kx))

d²/dx²(Bcos(kx)) = -k²(Bcos(kx))

Front 40

What is the 'true' wavefunction for a particle in a box?

Back 40

Linear combination of all potential solutions

=

ψ(x) = Asin(kx) + Bcos(kx)

Front 41

What are boundary conditions?

Back 41

They impose certain requirements on a wavefunction so that only specific solutions are acceptable. (this is why quantisation occurs)

Front 42

What are the boundary conditions for a particle in a box?

Back 42

ψ(x) = 0 when x=0 or x = L (or anywhere else in the classically forbidden region)

Front 43

What is the wavefunction of a particle in a box once ψ(x) = 0 when x = 0, has been considered?

Back 43

ψ(x) = A sin(kx)

because ψ(x) when x = 0 therefore B (from Bcos(kx)) must be zero because cos(0) = 1

Front 44

What is the wavefunction of a particle in a box once ψ(x) = 0 when x = L and 0, has been considered?

Back 44

ψ(x) = A sin(nπx/L) where n = 1,2 ...

because sinkx is only zero at x =L when kL is a multiple of π. (n = 0 is not a valid solution because then wavefunction would equal 0 at all x and then would not exist.

Front 45

What is k equal to after applying boundary condion ψ(x) = 0 when x = L?

Back 45

kL=nπ ∴ k = nπ/L

Front 46

What is a node when plotting a particle in the box solution?

Back 46

Where wavefunction cross zero. (at 0 and L wavefunction does not cross zero it merely ends at zero therefore these are not nodes)

Front 47

How many nodes are there for a particle in a box?

Back 47

N-1 nodes

Front 48

Do we need to normalise the particle in the box solution?

Back 48

yes

Front 49

How do we get energies from the normalised solution to the particle in a box wavefunction?

Back 49

Plug the wavefunction back into the Schrodinger equation or remember that k² = 2mE/ℏ² and k=nπ/L

∴E = ℏ² π²n²/2mL² = h²n²/8mL² where n = 1, 2....

Front 50

zero-point energy for a particle in a box?

Back 50

Where n = 1, (gonna have to work it out for yourself :P)

Front 51

What is a stationary state?

Back 51

A pure quantum state corresponding to a state with a single definite energy (instead of a quantum superposition of energy energies).

They are quantised

Front 52

What is the lowest energy called and why?

Back 52

It is called the zero-point energy, it is a non-zero energy therefore a particle at 0K still has energy

Front 53

What form does all the energy in a particle in a box take?

Back 53

Kinetic energy as V(x) is defined as 0.

Front 54

What is vibrational motion modled as ?

Back 54

A harmonic oscillator

Front 55

Hooke's law?

Back 55

F = -kx

where x is displacement

Front 56

What is the potential energy of a hormonic oscillator?

Back 56

kx²/2 [(1/2)* kx²]

Front 57

Why is reduced mass used?

Back 57

Changes a two-body problem into a one-body problem

Front 58

How is reduced mass calculated?

Back 58

Front 59

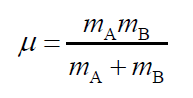
How do we write the Schrodinger equation for a harmonic oscillator system

Back 59

Front 60

What is the solution to the harmonic oscillator system?

Back 60

Ev = hve(v+1/2) where v =0, 1, 2...

where v in ve = greek 'nu' and is the molecular constant of vibrational frequency of the oscillator (units s⁻¹)

and v = italic v, and is the vibrational quantum number

Front 61

What is the vibrational frequencey of an oscillator defined as? (equation)

Back 61

ve = √(k/µ)

Front 62

How are the energy levels in an harmonic oscillator spaced?

Back 62

Energy levels are equally spaced unlike the particle in a box in which the energy levels become further apart an n increases.

Front 63

What is the zero-point energy for harmonic oscillator?

Back 63

Ezp = (1/2)*hve

=(hve/2)

Front 64

What does the solution of the harmonic oscillator schrodinger equation mean?

Back 64

A diatomic molecule can only vabrate at specific energies and these are called the vibrational levels (for that electronic state) hence why IR spectrum has well-defined lines

Front 65

What is the origin or quantisation in a hormonic oscillator system?

Back 65

The wavefunction penetrates into the classically forbidden region (V(x) > E) but only solutions that tend to zero but always curve away from the x axis will be allowed solutions

Front 66

What do the wavefunctions of the harmonic oscillator look like for its different energies?

Back 66

Front 67

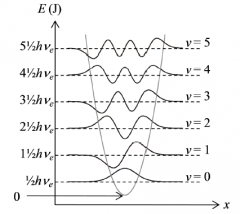
What does the plot of a high vibrational quantum number look like for a harmonic oscillator? + and its probability distribution

Back 67

Front 68

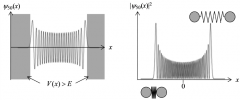
What does the plot of a probability distribution for a harmonic oscillator system tell us about the most probably inter-nuclear distance?

Back 68

The most probably inter-nuclear distance is either at the inner turning point or outer turning point

Front 69

Where is the greatest kinetic energy of a harmonic oscillator system?

Back 69

when the bond is at its equilibrium value (in the middle where curvature is at its greatest of a probability distribution plot. not at the turning points)

Front 70

What is the correspondence principle?

Back 70

States that in the limit of very large quantum number, the system is classical. This is th case for the macroscopic world.

Front 71

What is significant about ψ(x) at V(x) = E?

Back 71

The curvature must change sign as for V(x) < E curvature is towards the x axis but for V(x) > E the cuvarture is away from the x axis. (there is a point of inflection)

Front 72

What is quantum mechanical tunnelling?

Back 72

When a wavefunction enters the classically forbidden region it quickly tends to zero however if the 'barrier' has a finite width it is plausible that the wavefunction penetrating the barrier emerges on the other side before it has fallen to zero, in this case it has gone through the potentail barrier. The probability of tunnelling is small and only important for very light particles suchas electrons.

Front 73

How do we consider the rotation of a diatomic molecule in Quantum mechanics?

Back 73

model it as a particle on a ring

Front 74

How do we reduce the two-body problem of a rotation of a diatomic particle to a one-body problem?

Back 74

Transforming the system into a centre-of-mass system in which the reduced mass rotates on a ring of radius ro

Front 75

What assumption is made for a particle on a ring

Back 75

Free rotation

Front 76

If we assume free rotation for a particle on a ring system how does that help us?

Back 76

potential energy is zero (or constant)

Front 77

How is the schrodinger equation in the xy-plane expressed as for a particle on ring system?

Back 77

Front 78

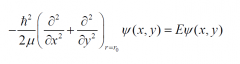
What is the polar coordinates version of the schrodinger equation in the xy-plane expressed as for a particle on a ring-system?

Back 78

Front 79

Back 79

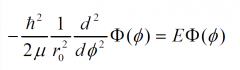
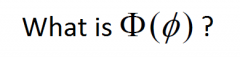
It is the angular wavefunction

Capital Phi (phi (some form))

Front 80

What is the angular wavefunction?

Back 80

A one dimenional function representing the properties of a particle on a ring system

Front 81

How do we express the schodinger equation for a particle on a string to allow us to solve it?

Back 81

Front 82

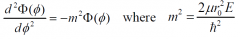
What are the solutions to the angular Schrodinger equation for a particle on a string?

Back 82

sine and cosine waves

Front 83

What is the superposed solution to the schrodinger equation?

Back 83

Front 84

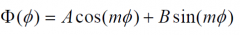
Boundary conditions for a particle on string?

Back 84

After one rotation the wave must be in phase with itself (heads and tails must match up)

Front 85

How do we apply the boundary conditions for a particle on a string?

Back 85

Front 86

Is ∅ = 0 allowed for particle on a ring? why?

Back 86

Yes, for ∅ = 0, into Φ(∅) = A cos(0) + Bsin(0) = A, therefore non-zero therfore can be normalised

Front 87

What is the quantisation defined for a particle on a string quantum system?

Back 87

Front 88

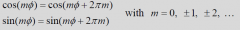
What quantum number is used for particle on a ring? + values?

Back 88

m, m= 0, ±1,±2...

Front 89

Quantised energy levels of a particle on a ring equation?

Back 89

Front 90

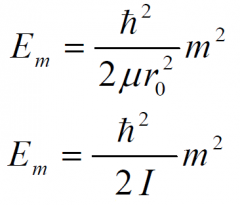
How many energy levels are there for every m for a particle on a ring?

Back 90

0 has 1, everything else has 2 (clockwise and anti clockwise)

Front 91

Zero point energy for a particle on a ring?

Back 91

When m = 0, E = 0, therefore no zero-point energy in rotation

Front 92

How many nodes does a wavefunction for a particle on a ring have?

Back 92

|m| nodes

Front 93

What is the particle on a sphere schodinger equation given in?

Back 93

spherical polar coodrinates (r, θ,∅)

Front 94

Angular wavefunctions for particle on a sphere?

Back 94

Front 95

Quantum numbers for a particle on a ring?

Back 95

l and ml

Front 96

What values can the particle on a sphere quantum numbers take?

Back 96

l = 0, 1 , 2 ....

ml = 0, ± 1, ± 2, ... ±l

Front 97

What is the equation for energy levels of the particle on a sphere?

Back 97

Front 98

How many ml quantum numbers are there for a l quantum number?

Back 98

2l + 1 ml quantum numbers

Front 99

Rotational constant of a molecule?

Back 99

Front 100

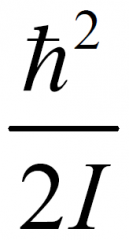
Which quantum number represents the quantum aspect of θ for a particle on a sphere?

Back 100

l

Front 101

Which quantum number represents aspect of ∅ for a particle on a sphere?

Back 101

ml

Front 102

How does nodes work with particle on a sphere?

Back 102

There are always l nodes total, however there are l - ml nodes on the z axis (θ spanse represented by l) and ml nodes on xy plane (∅ spance represented by ml )

Front 103

What is the potential energy of a H atom?

Back 103

Front 104

Radial wavefunction quantum numbers? (atomic orbitals)

Back 104

n and l

Front 105

Angular wavefunction quantum numbers? (atomic orbitals)

Back 105

l and projection of l onto the z-axis ml

Front 106

What are the quantum numbers for atomic orbitals? (+ allowed values)

Back 106

n - Principal quantum number (1, 2 ...)

l - angular momentum quantum number (0, 1 .. n-1)

ml - orientation (magnetic) quantum number (0, ±1,±2...±l)

Front 107

What equation defines the energy levels for atomic orbitals?

Back 107

Front 108

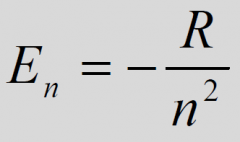
How many degenerate energy levels does each n state have?

Back 108

n x (2l + 1)

Front 109

How is the degeneracy different for non-hydrogen atoms?

Back 109

low l value wavefunctions are influenced by the core electrons

Front 110

Born oppenheimer Approximation?

Back 110

electrons move much faster than the nuclei hence electrons instantaneously adjust themselves to changes in the coordinates of the nuclei

Front 111

What is Ψmol made up?

Back 111

Ψel electronic wavefunction (which is an atomic orbitals)

Ψnucl nuclear wavefunction (which is a harmonic oscillator)

Front 112

How is a molecular potential energy curve obtained?

Back 112

solving schrodinger equation for the electrons at each internuclear separation

Front 113

What does the molecular potential energy curve look like?

Back 113

Front 114

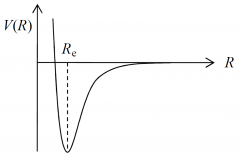
Why is a molecular potential energy curve called a potential energy curve?

Back 114

The nuclei are a fixed distance apart and thus have zero kinetic energy therefore all the energy must be potential

Front 115

What is Linear combination of Atomic Orbitals?

Back 115

we determine Ψ - from the superposition of atomic orbitals ψi as basis functions weighing each with a coefficient ci

Ψ = c1ψ1 + c2ψ2 ...

Front 116

in LCAO how do we find the probability of finding the electron in an orbital with the properties associated with atomic orbital ψi

Back 116

|ci|²

Front 117

What is the overlap densitiy in LCAO?

Back 117

|2Ψ1Ψ2|

Front 118

How is the overlap density obtained?

Back 118

Front 119

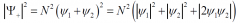
How does |2Ψ1Ψ2| vary with internuclear distance?

Back 119

overlap decreases with increasing internuclear distance

Front 120

What is the variation principle?

Back 120

method for estimating the energy and shape of a MO

Front 121

What assertion is the variation principle based on?

Back 121

The energy calculated using a trial wavefunction is always larger than the true energy

Front 122

How does one get the best approximation to the real wavefunction?

Back 122

using coefficients that lead to the lowest energy and using as many atomic orbitals as reasonably possible ( the more the better)

Front 123

Expectation value?

Back 123

used to find energy of the system

Front 124

How do we get the secular equations?

Back 124

Take partial derivative of expectation value with respect to the coefficients

Front 125

What are the secular equations for a diatomic molecule?

Back 125

Front 126

What does E, αa, αb, β, Sab mean from the secular equations for a diatomic molecule?

Back 126

E = total energy of MO

αa, αb = energies of the atomic orbitals ψa, ψb

β = resonance or interaction energy (energy gained through bonding)

Sab = overlap energy (spatial overlap of atomic orbitals)

Front 127

How do we know when β and Sab are non-zero (they are from secular equation)?

Back 127

Symmetry arguments- if ψa ψb are of the same symmetry then β<0 and Sab>0

Front 128

How do we simplify secular equations? (diatomic)

Back 128

assume negligible overlap of the atomic orbitals (Sab = 0)

Front 129

Simplified secular equation in matrix form?

Back 129

Front 130

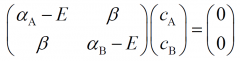
How do we know if there is a nontrivial solution to a matrix?

Back 130

Secular determinant = 0

Front 131

How do we get energies from secular determinant?

Back 131

set secular determinant to zero to give a polynomial in E then the roots are the energies

Front 132

What must we normalize on the LCAO wavefunction?

Back 132

only the coefficients because the wavefunctions are already normalised (because they are atomic orbitals)

Front 133

Huckel assuptions/approximations?

Back 133

-Consider only π orbitals which are formed from pz as these are responsible for most of the chemistry and spectroscopy

-α is the same for all atoms

-β is only relevant between neigbouring atoms otherwise β=0

-The overlap integrals are zero: S = 0

Front 134

What are the frontier orbitals?

Back 134

HOMO and LUMO

Front 135

How does chain length affect HOMO LUMO gap

Back 135

it decreases causing excitation energy to decrease giving longer wavelengths of light

Front 136

How do we find the probability of finding an electron localised on a specific atom in a specific orbital?

Back 136

square of the coefficient multiplied by number of electrons in orbital

Front 137

Total energy for a free rotation?

Back 137

Front 138

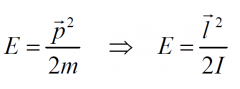
Angular momentum?

Back 138

Front 139

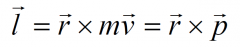
Moment of inertia I?

Back 139

equivalent to mass - is measure how resistant a object is to change

Front 140

Alternate angular momentum equation?

Back 140

Front 141

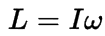
1D magnitude of the angular momentum?

Back 141

Front 142

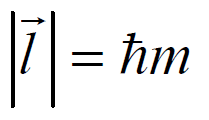
Angular momentum of an electron in an atomic orbit (particle on a sphere)?

Back 142

Front 143

Why is the orbital angular momentum quantised?

Back 143

The energy is quatised therefore angular momentum is quantised

Front 144

What does ml indicate about electron, what does l indicate about electron?

Back 144

ml quantum number indicates the directionality while l quantum number indicates the 'speed' of the electron in the orbit

Front 145

How do the orbital motion of electron affect each other?

Back 145

Generate magnetic field which influence (couples to) other electrons in an atom.

Front 146

Total angular momentum?

Back 146

Front 147

How many degenerate levels or projections are there for Total angular momentum L.

Back 147

2L+1

Front 148

Spin wavefunction for ms = +1/2 (spin up)?

Back 148

α

Front 149

Spin wavefunction for ms = -1/2 (spin down)?

Back 149

β

Front 150

Magnitude of a spin s = 1/2 particle?

Back 150

|s| = ℏ√s(s+1) =ℏ√1/2(1/2 +1) = 0.866ℏ

Front 151

Fluorescence vs phosphorescence?

Back 151

S = s1 + s2 . during excitation Fluorescence ∆S = 0, phosphorescence ∆S=1